Instructor Checklist for Piecewise Function Projects
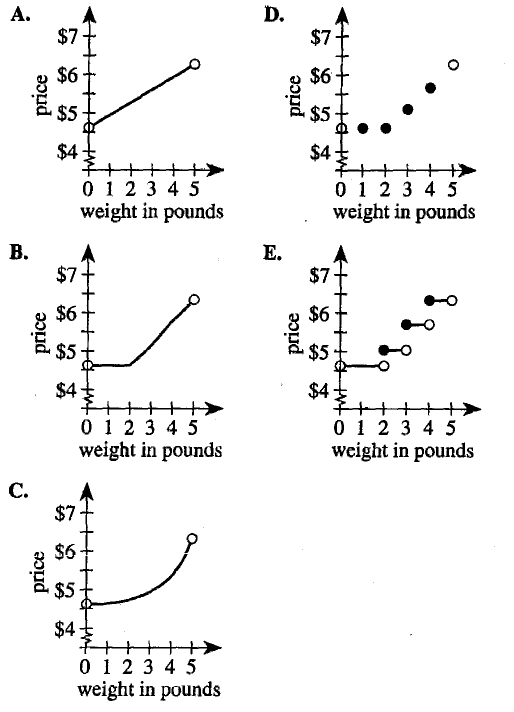
(1.) ACT The rental fee for the use of a reception hall is $500
To encoorage use of the hall's catering service, the hall's rental fee is reduced by $100 for each group of 20 meals ordered through the hall's catering service.
All 20 meals in a group must be ordered for each reduction in the rental fee.
One of the following graphs shows the hall's rental fee when 0 to 100 meals are ordered.
Which graph is it?

Let us represent the information using a Table of Values ... for 0 to 100 meals
Two variables: rental cost and number of meals
The rental cost depends on the number of meals
This implies that:
the rental cost is the dependent variable = y variable
the number of meals is the independent variable = x variable
The Table of Values is:
Based on the graphs, Options: F, G, and H satify the Table of Values
However, based on the wording in the question: All 20 meals in a group must be ordered for each reduction in the rental fee:
the correct answer is Option F because the increments must be in 20's only (no in-between values)
To encoorage use of the hall's catering service, the hall's rental fee is reduced by $100 for each group of 20 meals ordered through the hall's catering service.
All 20 meals in a group must be ordered for each reduction in the rental fee.
One of the following graphs shows the hall's rental fee when 0 to 100 meals are ordered.
Which graph is it?

Let us represent the information using a Table of Values ... for 0 to 100 meals
Two variables: rental cost and number of meals
The rental cost depends on the number of meals
This implies that:
the rental cost is the dependent variable = y variable
the number of meals is the independent variable = x variable
The Table of Values is:
| Number of meals | Rental cost ($\$$) |
|---|---|
| $x$ | $y$ |
| $0$ | $500$ |
| $20$ | $400$ |
| $40$ | $300$ |
| $60$ | $200$ |
| $80$ | $100$ |
| $100$ | $0$ |
Based on the graphs, Options: F, G, and H satify the Table of Values
However, based on the wording in the question: All 20 meals in a group must be ordered for each reduction in the rental fee:
the correct answer is Option F because the increments must be in 20's only (no in-between values)
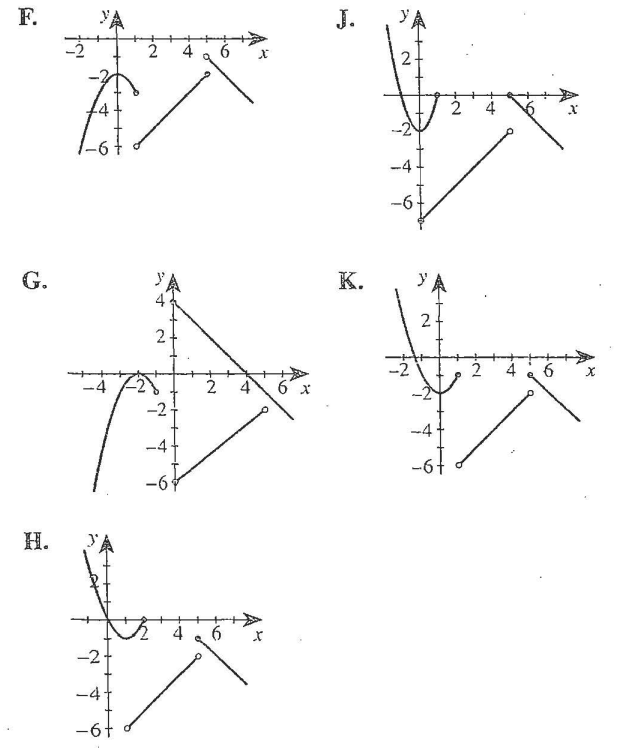
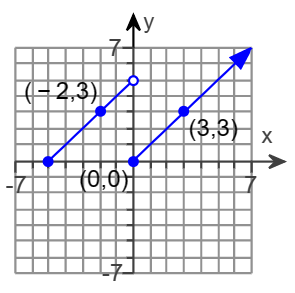
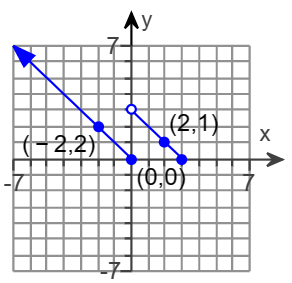
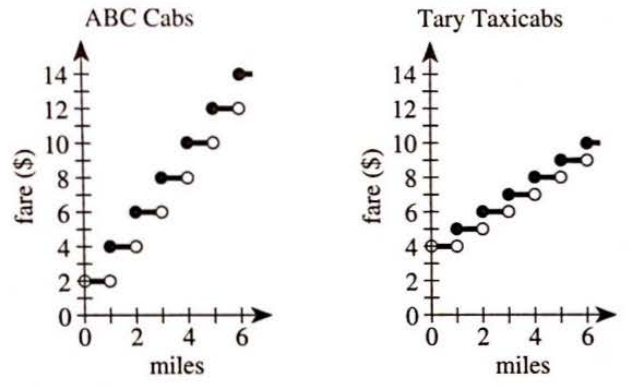
(2.) ACT ABC Cabs and Tary Taxicabs both have an initial fare of a while number of dollars for 1 passenger.
The fare increases a whole number of dollars at each whole number of miles traveled.
The graphs below show the 1-passenger fares, in dollars, for both cab companies for trips up to 6 miles.
When the fares of the 2 cab companies are compared, what is the cheaper fare for a 5-mile trip?
$ F.\;\; \$ 8 \\[3ex] G.\;\; \$ 9 \\[3ex] H.\;\; \$ 10 \\[3ex] J.\;\; \$ 11 \\[3ex] K.\;\; \$ 12 \\[3ex] $
The value in the y-axis (the fare) for the closed circle value in the x-axis (the miles)
$ For\;\;a\;\;5-mile\;\;trip: \\[3ex] ABC\;\;Cabs:\;\;fare = \$12 \\[3ex] Tary\;\;Taxicabs:\;\;\; fare = \$9 \\[3ex] Cheaper\;\;fare = \$9 $
The fare increases a whole number of dollars at each whole number of miles traveled.
The graphs below show the 1-passenger fares, in dollars, for both cab companies for trips up to 6 miles.
When the fares of the 2 cab companies are compared, what is the cheaper fare for a 5-mile trip?

$ F.\;\; \$ 8 \\[3ex] G.\;\; \$ 9 \\[3ex] H.\;\; \$ 10 \\[3ex] J.\;\; \$ 11 \\[3ex] K.\;\; \$ 12 \\[3ex] $
The value in the y-axis (the fare) for the closed circle value in the x-axis (the miles)
$ For\;\;a\;\;5-mile\;\;trip: \\[3ex] ABC\;\;Cabs:\;\;fare = \$12 \\[3ex] Tary\;\;Taxicabs:\;\;\; fare = \$9 \\[3ex] Cheaper\;\;fare = \$9 $